Please be sure to answer the questionProvide details and share your research! 3,304 2 i think you dropped a sign somewhere, but i would go as follows x 2 y 2 = x 2 y 2 0 = x 2 y 2 ( (xy) (xy)) = x 2 xy xy y 2 but as I think Mark was pointing out, all the steps you took working from RHS to LHS are perfectly valid in \(\displaystyle Prove \lim_{(x,y) \to (0,0)}\frac{2xy^2}{x^2y^2} = 0\) There are probably many ways to do this, but my teacher does it a certain way and I would like to learn his way first (although I am also interested in other techniques–perhaps
Prove That If X Y Z 0 Then Xy Yz Zx 0
Prove (x+y)^2=x^2+2xy+y^2
Prove (x+y)^2=x^2+2xy+y^2-MarchLight MarchLight Mathematics High School answered How to solve (xy)^2 (x^2 2xyy^2) 1 See answer my question is, there is no equal sign so you can't prove it Since the first sign is negative both signs must be negative The factors are (xy)(xy) or (xy)^2 Check by FOIL Firsts (x)(x) = x^2 Outers (x)(y) = xy Inners (y)(x) = xy Lasts (y)(y) = y^2 combine the middle terms (xy)(xy) = 2xy x^22xyy^2



Find Dy Dx When X And Y Are Connected By The Relation X2 Y2 2 Xy Studyrankersonline
First, you want to expand the equation so it'll be x^22yxy^2=x*22xyy^2 Then you subtract y^2 from both sides x^22yxy^2y^2=x*22xyy^2y^2 After that, you simplify x^22yx=x*22xyDetecting a perfect square 12 x2 2xy y2 is a perfect square It factors into (xy)• (xy) which is another way of writing (xy)2 How to recognize a perfect square trinomial • It has three terms • Two of its terms are perfect squares themselves • The remaining term is twice the product of the square roots of the other two termsSolution for x^22xyy^2=0 equation Simplifying x 2 2xy y 2 = 0 Reorder the terms 2xy x 2 y 2 = 0 Solving 2xy x 2 y 2 = 0 Solving for variable 'x' Factor a trinomial (x 1y)(x 1y) = 0 Subproblem 1 Set the factor '(x 1y)' equal to zero and attempt to solve Simplifying x 1y = 0 Solving x 1y = 0 Move all terms containing x to the left, all other terms to the right
Gold Member 4,540 581 (xy) 2 = x 2 2xy y 2 >= 0 You know that already So x 2 xy y 2 >= xy If x and y are both positive, the result is trivial If x and y are both negative, the result is also trivial (in both cases, each term in the summation is positive) Foil out the left side of the equation (xy) (xy)=x^2xyyxy^2=x^22xyy^2 Hope this helps! LHS (xy)^2 = x^2 2xy y^2 RHS (xy)^2 4xy = (x^2 2xy y^2) 4xy =x^2 2xy y^2 4xy =x^2 2xy 4xy y^2 =x^2 2xy y^2 Hope, this may help you Mark as Brainliest, plz kaypeeoh72z and 44 more users found this answer helpful
That is, (1 xAbove proof would be \If 2xy xy p xy then 0 (x y)2," which is true but not what we want to prove (we want to prove the converse) Remember that proofs are meant to be read If you cannot read your proof aloud so that your logic is understandable, it needs work Also for fun, one student found a nice proof of the inequality that also involves squaring both sides let A = 2xy xy and let B =Factor 2x^2xyy^2 2x2 − xy − y2 2 x 2 x y y 2 For a polynomial of the form ax2 bx c a x 2 b x c, rewrite the middle term as a sum of two terms whose product is a⋅c = 2⋅−1 = −2 a ⋅ c = 2 ⋅ 1 = 2 and whose sum is b = −1 b = 1 Tap for more steps Reorder terms 2 x 2 − y 2 − x y 2 x 2 y 2 x y




Is Math X Y 2 X 2 Y 2 Math Or Math X 2 2xy Y 2 Math Quora




Ex 2 5 9 Verify I X3 Y3 X Y X2 Xy Y2 Ex 2 5
Free PreAlgebra, Algebra, Trigonometry, Calculus, Geometry, Statistics and Chemistry calculators stepbystepYou are being tasked to prove A > B A is x >= 0 and y >= 0 B is (xy)/2 >= sqrt(xy) If you are studying proofs and real numbers, I doubt that at this point you need to prove that (as picado said) z 2 >= 0 for z real number If you have seriously not encountered this yet, then you must do so Thanks for contributing an answer to Mathematics Stack Exchange!




Implicit Differentiation Advanced Example Video Khan Academy




How To Simplify Math Frac X 2 2xy Y 2 X 2 2xy Y 2 X 2 Y 2 2 Math Quora
Click here👆to get an answer to your question ️ Verify x^3 y^3 = (x y)(x^2 xy y^2) using some non zero positive integers and check by actual multiplication Can you call theses as identities? \(\frac{dy}{dx} = \frac{y^2x^2}{2xy},\) This is a Homogeneous DE Therefore, put y = vx and \(\frac{dy}{dx}\) = v x \(\frac{dv}{dx}\) to convert itConsider x 2 y 2 x y − 2 2 x y as a polynomial over variable x Find one factor of the form x^ {k}m, where x^ {k} divides the monomial with the highest power x^ {2} and m divides the constant factor y^ {2}y2 One such factor is xy1 Factor the polynomial by dividing it by this factor
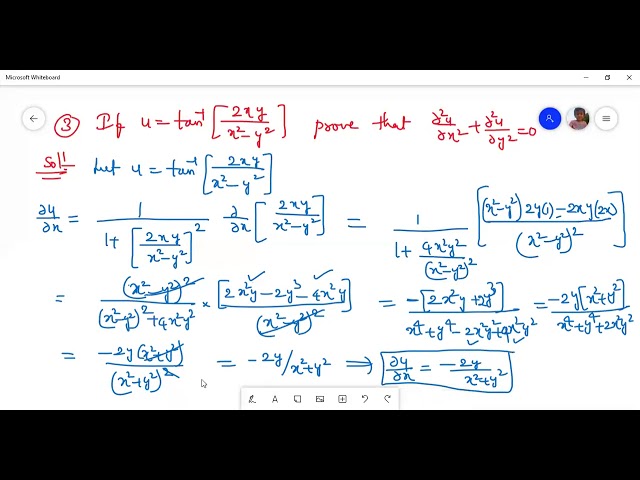



Problems On Partial Differentiation U Log X 2 Y 2 Z 2 U Tan 1 2xy X 2 Y 2 Z F X Ay Q X Ay Youtube




Keep Calm I Have Algebraic Proof X 2 2xy Y 2 X 2 2xy Y 2 X 4 4xy Y 4 Keep Calm And Posters Generator Maker For Free Keepcalmandposters Com
2 x is a function of y 3 x and y are both functions of some variable (call it t) 4 x is a constant 5 y is a constant Setup Assuming y is a function of x 2 Assuming x is a function of y It's just the same steps as number 1, but with y and x replaced, sinceCompute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historyI need to prove using the $ \epsilon\delta$ definition that $ $ \lim_{(x,y)\to(7,2)} x^2 y^2xy=39$ $ So I begin by rewriting the absolute value $ $ \mid x^2 y^2 – xy 39 \mid = \mid (x7)(x7) (y2)(y2) – (x7)(y2) 2(x7) 7(y2)\mid$ $ Using the triangle inequality, we have $ $ \leq \mid x7\mid Continue reading "Proving that $\lim_{(x,y)\to(7,2)} x^2 y^2xy=39$ using only
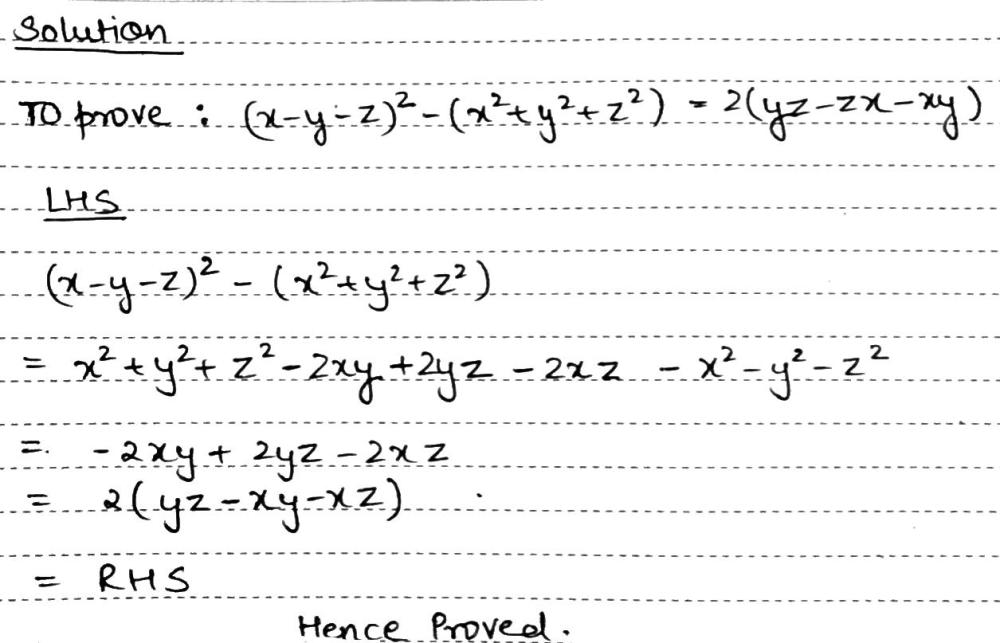



Prove X Y Z 2 X 2 Y 2 Z 2 2 Yz Zx Xy Snapsolve
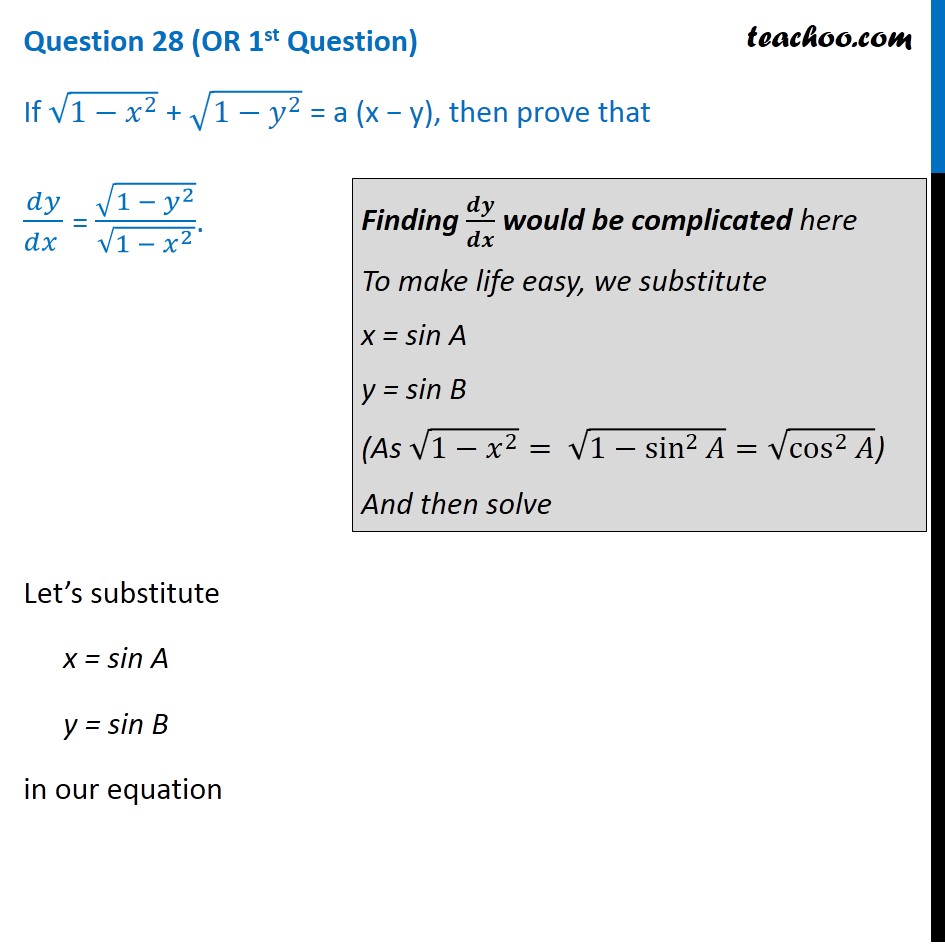



If 1 X 2 1 Y 2 A X Y Then Prove That Dy Dx
But avoid Asking for help, clarification, or responding to other answersPolynomial identities (short multiplication formulas) (x y) 2 = x 2 2xy y 2 (x y) 2 = x 2 2xy y 2 Example 1 If x = 10, y = 5a (10 5a) 2 = 10 2 2·10·5a (5a) 2 = 100 100a 25a 2 Example 2 if x = 10 and y is 4 (10 4) 2 = 10 2 2·10·4 4 2 = 100 80 16 = 36 The opposite is also true 25 a 4a 2 = 5 2Steps Using the Quadratic Formula { x }^ { 2 } { y }^ { 2 } 2xy=0 x 2 y 2 2 x y = 0 All equations of the form ax^ {2}bxc=0 can be solved using the quadratic formula \frac {b±\sqrt {b^ {2}4ac}} {2a} The quadratic formula gives two solutions,




Ex 2 5 12 Verify That X3 Y3 Z3 3xyz 1 2 Ex 2 5




Verify That X 3 Y 3 Z 3 3xyz 1 2 X Y Z X Y 2 Y Z 2 Z X 2 Math Polynomials Meritnation Com
F(x;y)=œ xy(x2−y2) x2y2 (x;y)≠(0;0) 0 (x;y)=(0;0) Note fis continuous, (by computing lim(x;y)→(0;0) of the formula above, eg using polar coorinates) (a) Find f x and f y when (x;y)≠(0;0) Away from (0;0);fcan be di erentiated using the formula de ning it, as @f @x (x;y)= (x2 y2)y(x2 −y2)2x2y−2x2y(x2 −y2) (x 2y)2;I SHALL SHOW YOU THE FIRST TRY DOING OTHERS IN A SIMILAR WAYIF IN DIFFICULTY COME BACKClick here👆to get an answer to your question ️ If 2costheta sintheta = x & costheta 3sintheta = y , Prove that 2x^2 y^2 2xy = 5



Prove The Inequality X X 1 Y Y 1 Xy 1 X X 1 Y Y 1 Xy 1




Worked Example Implicit Differentiation Video Khan Academy
Prove sqrt(xy) is less than or equal to (xy)/2 for all positive values of x and y Well if x,y positive then wlog x=a^2, y=b^2 means you want to prove 2abImage transcriptions ( 2 x y cos x 2 _ 2 x y 1 ) dx ( sin x 2 x 2 ) dy = 0 first order linear ordinary differential Equates A first order linear ODE has the form y' ( 4) p(xjy = 9 ( x ) let y be the dependent variable Divide by dx If zug coslay any ( sin 123 29 dy da sub stitude with yo rewrite this into form of linear ODE y 2 2 ( cos (29 1 ) y 1 = 212 sin (my sin 107Question Prove Y(x)=sqr Rt(x^2cx) Is The General Solution Of Differential Equation Dy/dx=(y^2x^2)/(2xy) This problem has been solved!




Let U Xy X Y Show That X2 2u X2 2xy 2u X Chegg Com




Mathematics Class 9th Chapter 4 Solution
(xy) is proportion to (xy) Therefore, (xy)=k(xy) or , (xy)^2 = k^2(xy)^2 or , x^2y^22xy = k^2(x^2y^22xy) or (x^2y^2) k^2(x^2y^2) = 2xy 2k^2Thanks answered Guest 1 f (x)=x²10x16 use the formula to find the vertex = (b/2a, f (b/2a)) , here in the above equation a=1 (as, a> 0 the parabola is open upward), b=10 by putting the values b/2a = 10/2 (1) = 5Answer to Consider F and C below F(x, y) = (5 2xy^2)i 2x^2y j, C is the arc of the hyperbola y = 1/x from (1, 1) to (3, 1/3) Find a




Prove That 2x2 2y2 2z2 6xyz X Y Z X Y 2 Y Z 2 Z X 2 Hence Evaluate 2 7 3 2 9 3 2 13 3 6 7 9 13 Maths Number Systems Meritnation Com




If Log X Y 2 1 2 Log X Log Y Prove That X 2 Y 2 6xy Brainly In
Question Geometricly prove that (xy)^2 = x^22xyy^2 (xy)^2 = x^22xyy^2 (xy)(xy) = x^2y^2 Answer by venugopalramana(3286) (Show Source) You can put this solution on YOUR website!∴ y^2 = (2x)^2 = (x2)^2 Also, x^2 y^2 = x^2 2xy y^2 2xy = (x y)^2 2xy = 2^2 2xy = 4 2x (2x) = 4 4x x^2 = (x 2)^2 So x^2 y^2 (x^2 y^2) = x^2 (x2)^2 (x 2)^2 = x^2 (x2)^4 Question Prove that \( u=x^2y^2\frac{1}{2}\ln{\left(x^2y^2\right)}\) is harmonic and find it's harmonic conjugate\\ Solution A function \(u\left(x, y\right




Cse115 Engr160 Discrete Mathematics 02 07 12 Ppt Download
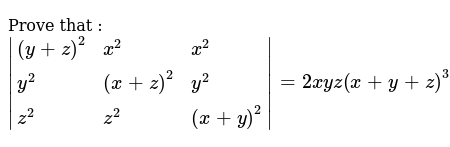



Prove That Y Z 2 X 2 X 2 Y 2 X Z 2 Y 2
যদি `x=1&y=3` তারপর পরিচয় প্রমাণ `(xy)^2=x^22xyy^2` JEE Main 21 4th session starts from Aug 26, application last date extendedAnswer to Prove that 2xy\ dx(x^2y^2)\ dy=0 By signing up, you'll get thousands of stepbystep solutions to your homework questions You can If x/ sin thet 2 y 2 /b 2 =2 Get the answers you need, now!
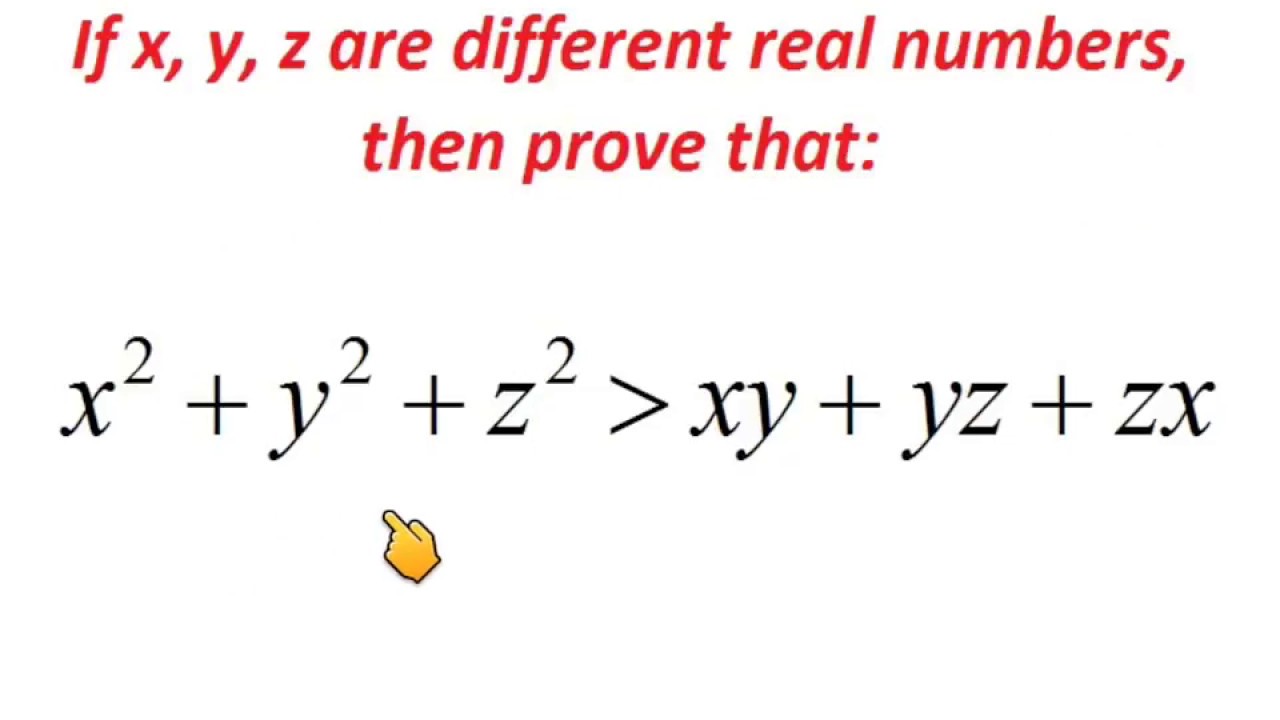



X 2 Y 2 Z 2 Greater Than Xy Yz Xz Proof Important For Iit Jee Nda Scra Sat Competitive Exams Youtube




If X2 Y2 25xy Then Prove That 2 Log X Y 3log3 Log X Log Y Brainly In
Then it follows that lcm (3,4,5) xyz To prove each of the three statements above, consider the quadratic residues mod 3, 4, or 5 a 2 = r (mod 3) List out all of the possibilities for r Since you know x 2 y 2 = z 2 (mod 3), try to rule out some possibilities and see where that takes you 1If u = x 2 − y 2, v = 2 x y a n d z = f ( u, v) prove the following written 50 years ago by shailymishra30 ♦ 330 modified 14 months ago by sanketshingote ♦ 570 ( ∂ z ∂ x) 2 ( ∂ z ∂ y) 2 = 4 u 2 v 2 ( ∂ z ∂ u) 2 ( ∂ z ∂ v) 2 partial differentiation ADD COMMENTWe think you wrote (x^22xyy^2)/(x^2−y^2)*(5x^2−3xy−2y^2)/(2x^2−3xy−5y^2) This deals with factoring multivariable polynomials




Math Formula X Y Math Formulas




Show That The Equation 5x4 3x2y2 2xy3 Dx 2x3y 3xy2 5y4 Dy 0 Is An Exact Differential Equation Find Its Solution Mathematics 2 Question Answer Collection
(x;y)!(0;0) 2xy x2 2y2 = lim x!0 0 x2 = 0 Approaching (0;0) along the yaxis (x = 0), lim (x;y) !(0;0) 2xy x2 2y2 = lim y 0 0 2y2 = 0 Approaching (0;0) along the line y = x, lim (x;y)!(0;0) 2xy x2 2y2 = lim x!0 2x2 3x2 = 2 3 The limit does not exist Example Find the limit lim (x;y)!(0;0) x2y x4 y2 if it exists Approaching (0;0We want x y so the simplest approach is to find x and then y and then simply multiply the two together We have two equations (essential for two unknowns) one linear, x − y = 7, and one which is the equation of a circle with its centre at (0, 0) and a radius of 5The easiest method is to rearrange the linear equation in terms of x or y and then substitute it into the circle equation= (x 2 y 2 2xy) (z 2 x 2 2zx) (y 2 z 2 2yz) = (x y) 2 (z x) 2 (y z) 2 Since square of any number is positive, the given equation is always positive



Http Www Math Kent Edu Soprunova f16 Inequalities16 Pdf
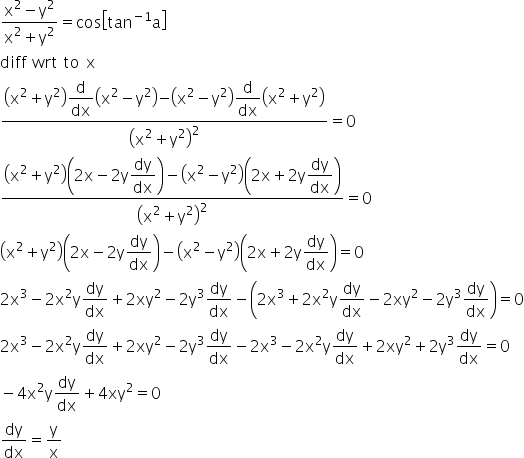



If Cos 1 X2 Y2 X2 Y2 Tan 1 A Prove That Dy Dx Y X Mathematics Topperlearning Com K6vhyqq
PreAlgebra Examples Expand (x−y)(x2 −2xyy2) ( x y) ( x 2 2 x y y 2) by multiplying each term in the first expression by each term in the second expression Simplify each term Tap for more steps Multiply x x by x 2 x 2 by adding the exponents How to solve (xy)^2 (x^2 2xyy^2) Get the answers you need, now!Prove That `Tan^1 (2ab)/(A^2b^2)Tan^1 (2xy)/(X^2y^2)=Tan^1 (2alphabeta)/(Alpha^2beta^2),` Where `Alpha=Axby And Beta=AyBx`




1 Evaluate The Limit X Y 2 Lim X Y 3 1 Chegg Com



Www Math Ucla Edu Mt 131a 1 02s 131a Hw Sol Pdf
Let x;y 2R Observe that 0 (x y)2 = x2 2xy y2 Adding 2xy to both sides of the previous inequality we obtain x2 y2 2xy which is precisely what we wanted to prove 2 Let x > 1 and n be a positive integer Prove Bernoulli's inequality (1 x)n 1 nx Proof This proof will be done by induction on n 1 Since (1x)1 = 1x for any x 2R, the inequality holds when n = 1 Suppose now that the inequality holds for some n = k 1; Show that the differential equation (xy)dy/dx=x2y,is homogeneous and solve it asked in Mathematics by Nisa ( 597k points) differential equations



Find Dy Dx When X And Y Are Connected By The Relation X2 Y2 2 Xy Studyrankersonline




Prove That X 3 Y 3 Z 3 3xyz 12 X Y Z X Y 2 Y Z 2 Z X 2




If 2x 3y 12z Show That X 2y Z Xy Brainly In




Direct Proof And Counterexample I Ppt Download



19 Evaluation Of Proofs This Type Of Exercise Will Chegg Com




Prove That The Points Of Intersection Of The Line X Y 2 With The




Q 1 Prove That 2x 3 2y 3 2z 3 6xyz X Y Z Formula X Y 2 Y Z 2 Z X 2 Maths Polynomials Meritnation Com



Search Q X 5e2 2b2xy 2by 5e2 Graph Tbm Isch




X2 2xy Y2 Dx X Y 2dy 0 Brainly In




2 A X Y B X2 Xy Y2 C X4 X2y2 Y4 D 81x4 Y4 22x2y2 Gauthmath



How To Prove That Math X 2 Y 2 Z 2 Xy Yz Zx Math Is Always Positive Quora



If X 2 2xy 3y 2 1 Show That Y 2 2 X 3y 3 Sarthaks Econnect Largest Online Education Community




How Do I Prove That X 2 Y 2 X Y 2 2xy Geometrically Intuitively Mathematics Stack Exchange




X3 Y3 Z3 3xyz 1 2 X Y Z X Y 2 Y Z 2 Z X 2 Novocom Top




When X 2 Y 2 T 1 T And X 4 Y 4 T 2 1 T 2 Then How Do You Prove That Dy Dx 1 X 3y How Would You Solve This Quora



Find The Sum Of The Geometric Series Sequences And Series Maths Class 11



Prove That If X Y Z 0 Then Xy Yz Zx 0




Get Answer Proof By Cases Use A Proof By Cases To Show That X 2 2xy Y 2 Transtutors




How Can I Prove That Xy Leq X 2 Y 2 Mathematics Stack Exchange



If X Seca Sina And Y Seca Sina Prove That 2 X Y 2 X Y 2 2 1
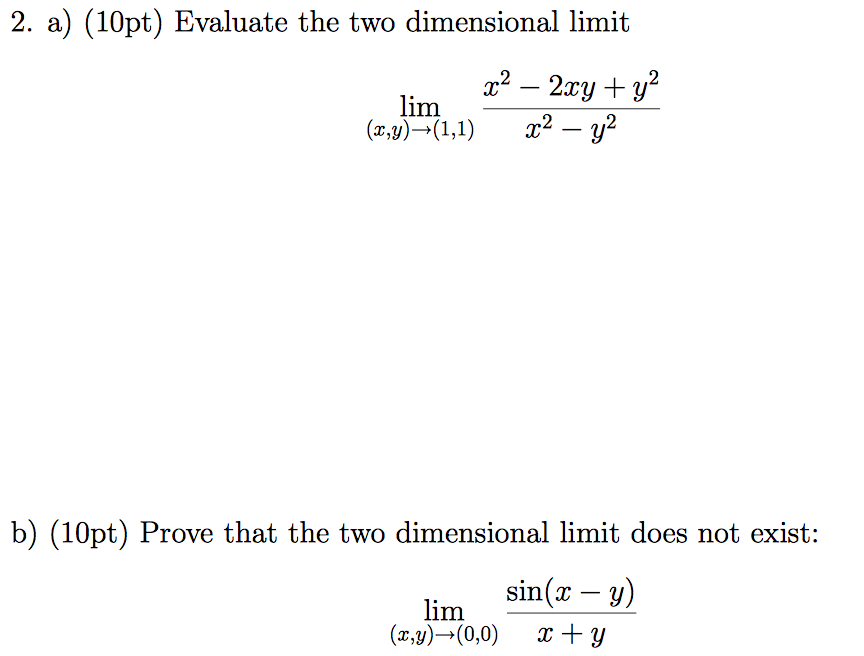



Evaluate The Two Dimensional Limit Limit X Y Chegg Com




Ex 9 5 15 Class 12 Find Solution 2xy Y 2 2x 2 Dy Dx 0 When



If U Log X 2 Y 2 Xy Then X U X Y U Y Is A 0 B U C 2u Sarthaks Econnect Largest Online Education Community




Show Function F X Y X 2 Y 2 2xy Is 1 1 By Inverse Function Theorem Mathematics Stack Exchange



2




If Math 3x 4y Sqrt Xy Math How Can You Prove That Math X 2 Y 2 Xy Math Quora




Ipe Material Notes



Q 3 Find The Integrating Factor And Solve The Exact Solution Consider The Differential Equation E X Y Cosy Cosx 2e 2x Y Dx Sinx E X Y Siny 3y 2e Y Dy Q 5 Prove Socratic



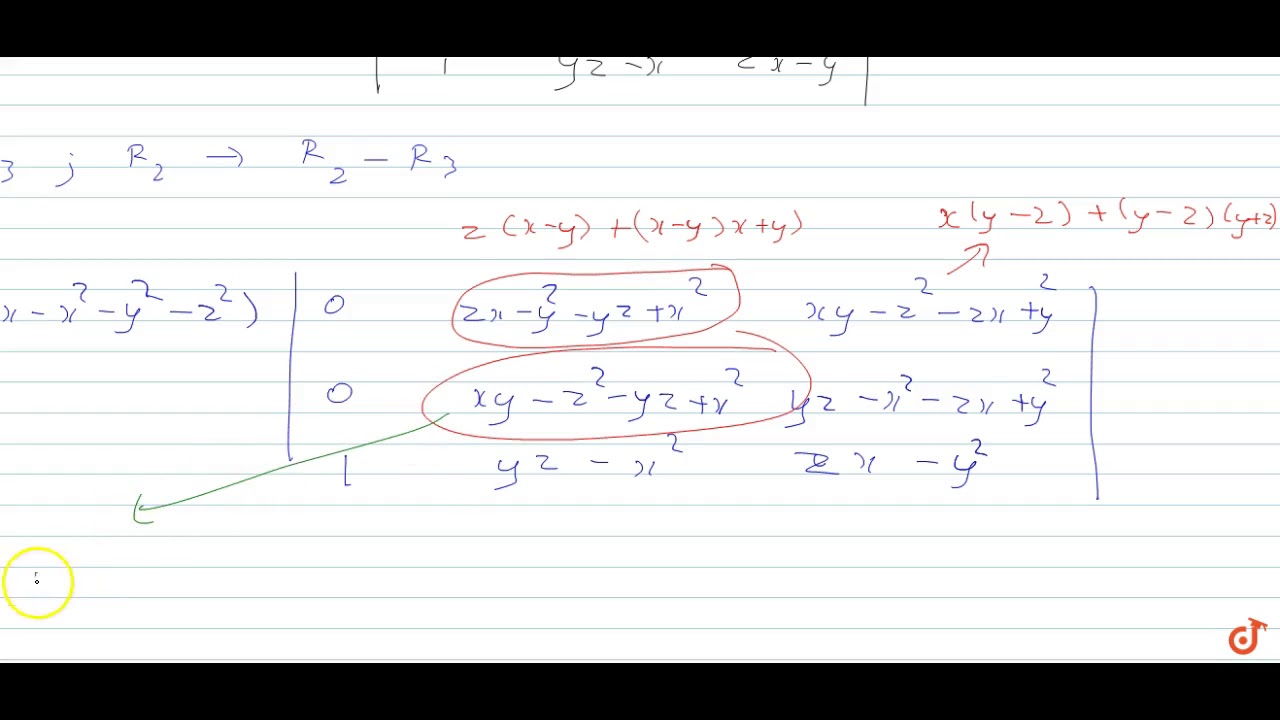
Using Properties Of Determinants Prove That Y X 2 Xy Zx Xy X Z 2 Yz Xz Yz X Y 2 2xyz X Y Z 3 Sarthaks Econnect Largest Online Education Community
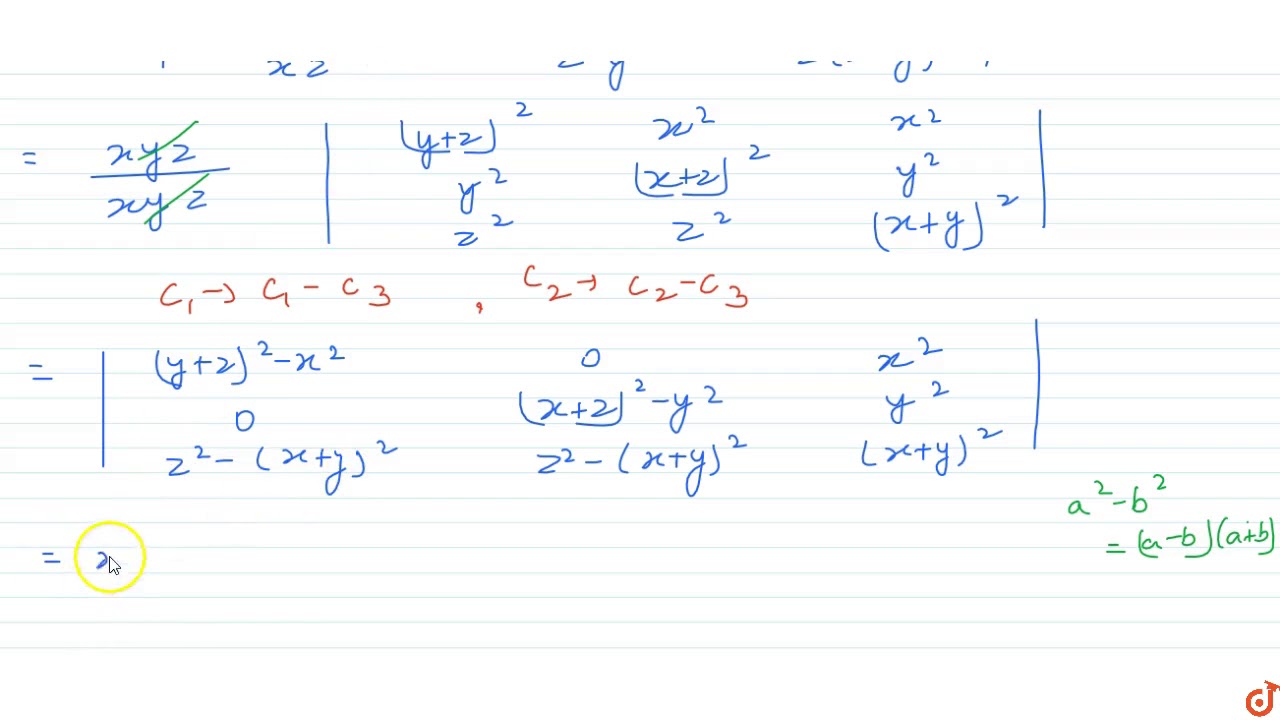



Prove That Y Z 2 Xy Zx Xy X Z 2 Yz Xz Zy X Y 2 Xyz X Y Z 3 Youtube




Prove That The Curve X 2 Y 2 X 2y 0 And Xy 2x Y 0 Intersect Ort
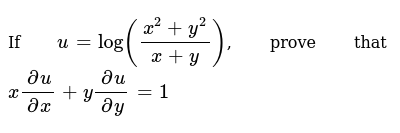



If U Log X 2 Y 2 X Y Prove That X Delu Delx Y Delu D



Does Sqrt X 2 Y 2 X Y Brilliant Math Science Wiki




B Prove The Following Determinant By Using Propert Gauthmath



If Math X Y 2 Text And X 2 Y 2 2 Math What Is The Value Of Math Xy Math Could You Break It Down For Me Quora



Http Www Uuooidata Org Data Course Sns S025 Week 3 Pdf



Prove That Det Yx X 2 Zx Y 2 Xy Z 2 Zx Y 2 Xy Z 2 Yz X 2 Xy Z 2 Yz X 2 Zx Y 2 Is Divi Youtube



Prove That 2x3 2y3 2z3 6xyz X Y Z X Y 2 Y Z 2 Z X 2 Hence Evaluate 2 7 3 2 9 3 2 13 3 6 7 9 13 Mathematics Topperlearning Com V780o2jj




Solved Prove By Hand That F R Right Arrow R X Y Ri Chegg Com




2 A X Y B X2 Xy Y2 C X4 X2y2 Y4 D 81x Y 22x3y2 E Gauthmath
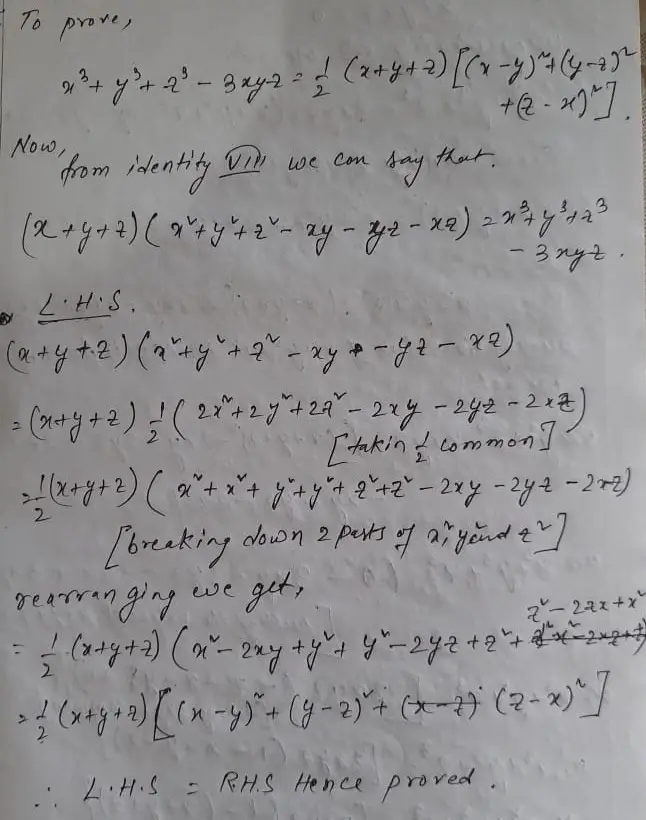



Telangana Scert Class 9 Math Solution Chapter 2 Polynomials And Factorisation Exercise 2 5




Using Properties Of Determinant Prove That 1 X Y X 2 Y 2 1




Slides Show



2 A X Y B X2 Xy Y2 C X4 X2y2 Y4 D 81x Y 22x3y2 E Gauthmath




D D1rect1on Complete The Give See How To Solve It At Qanda
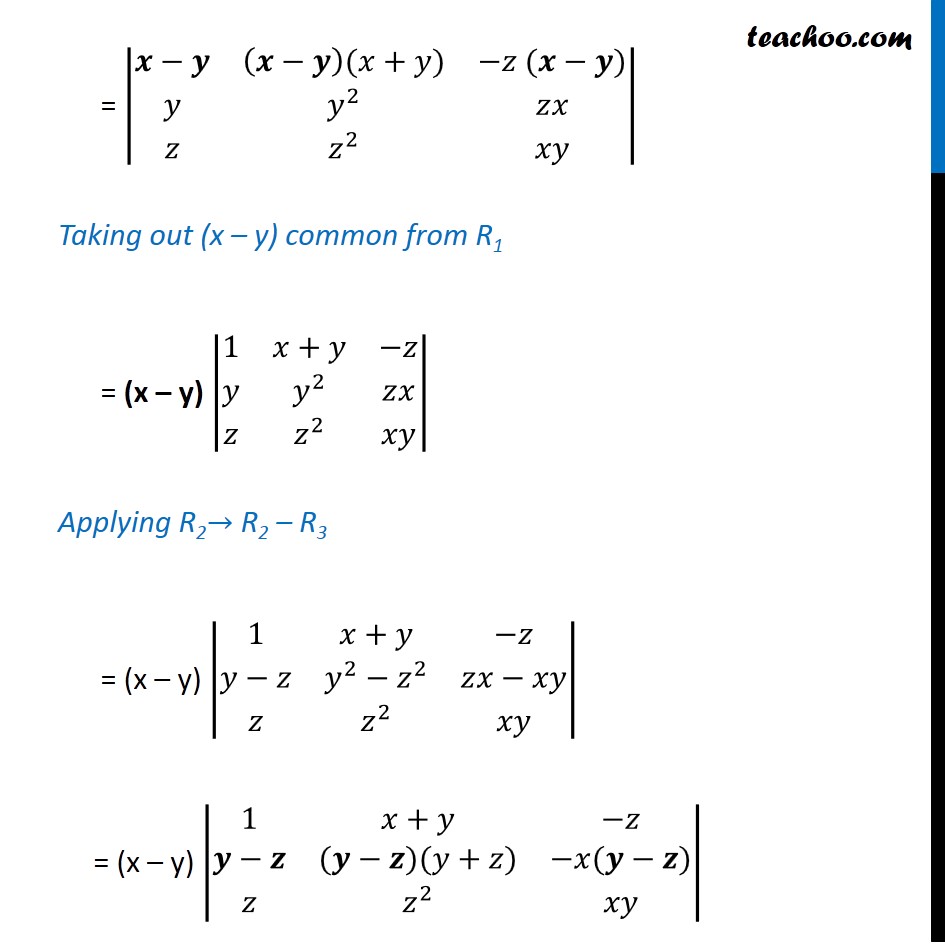



Ex 4 2 9 Show That X X2 Yz Y Y2 Zx Z Z2 Xy X Y Y Z




How Can I Prove That Xy Leq X 2 Y 2 Mathematics Stack Exchange




Algorithm For X 2 Xy Y 2 Z 2 With The Solution 1 1 1 That Download Scientific Diagram



A B 2



How To Prove X 2 Y 2 Xy If X Y X Y Quora




If X Y Z 0 Then Prove That X 2 Yz Y 2 Zx Z 2 Xy 3 Brainly In




If 2 X 3 Y 12 Z Then Prove That Xy Z X 2y Mathematics Topperlearning Com 8v1liiww




If X Y Z 0 Then Prove That The Square Of The Value X Y 2 Xy Y Z 2 Yz Z X 2 Zx 9



Www Math Ucla Edu Mt 131a 1 02s 131a Hw Sol Pdf



Prove That Yz X 2 Zx Y 2 Xy Z 2 Zx Y 2 Xy Z 2 Yz X 2 Xy Z 2 Yz X 2 Zx Y 2 Is Divisible By X Y Z And Hence Find The Quotient Sarthaks Econnect Largest Online Education Community



1




Find The Sum Of Following Ap 2 X Y 2 X2 Y2 X Y 2 Up To N Terms Brainly In



Prove Using Properties Of Determinants X Y 2z X Y Z Y Z 2x Y Z X Z X 2y 2 X Y Z 3 Sarthaks Econnect Largest Online Education Community



Prove X 2 Y 2 2xy And X 2 Y 2 Z 2 1 3



If U X 2tan 1 Y X Y 2tan 1 X Y Prove That 2u Y X X 2 Y 2 X 2 Y 2 Sarthaks Econnect Largest Online Education Community




Let E X 3y 2x Y Y X Y Er 1 Prove That E Chegg Com




How Do I Prove That X 2 Y 2 X Y 2 2xy Geometrically Intuitively Mathematics Stack Exchange




Pdf A Result Of Commutativity Of Rings




Prove That The Angle Between The Straight Lines Joining The Origin To The Intersection Of The Straight Line Y 3x 2 With The Curve X 2 2xy 3y 2 4x 8y 11 0 Is Tan 1 2 2 3




Xy X 1 Y Y 0 Novocom Top
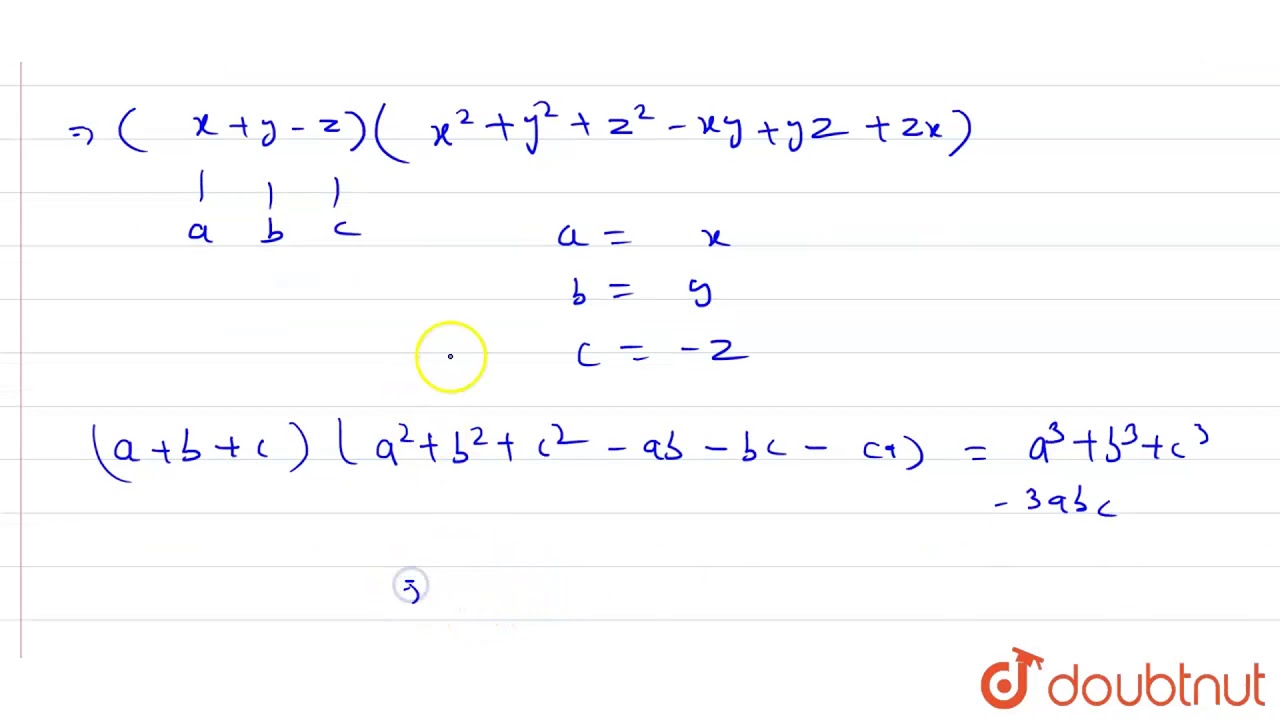



Find The Product X Y Z X 2 Y 2 Z 2 Xy Yz Zx Youtube




Assignment No



How To Prove X 2 Y 2 Xy If X Y X Y Quora
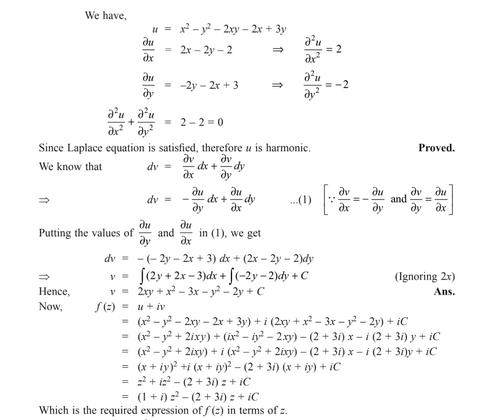



Prove That U X 2 Y 2 2xy 2x 3y Is Harmonic And Find Harmonic Conjugate V Edurev Iit Jam Question



Multiply X2 4y2 Z2 2xy Xz 2yz By Z X 2y Studyrankersonline
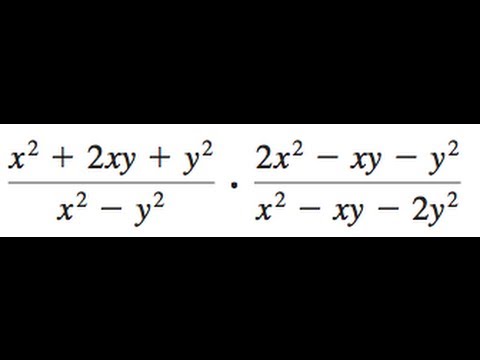



X 2 2xy Y 2 X 2 Y 2 2x 2 Xy Y 2 X 2 Xy 2y 2 Youtube




If Y 1 M Y 1 M 2x Show That X2 1 Y2 Xy1 M2 Y Mathematics Topperlearning Com 31vqi0ff



Prove That The Curves Xy 4 And X2 Y2 8 Touch Each Other Studyrankersonline




If X2 Y2 2 Xy Find Dy Dx Mention Each And Every Step Mathematics Topperlearning Com Gwvwfskk




If 2 X 2 Y 2 X Y Then Dy Dx Is Equal To 2 Y 2 X




If U Log X 2 Y 2 Z 2 Verify 2u X Y 2u X Y



What Is A Mathematical Proof An Aspiring Rationalist S Ramble



0 件のコメント:
コメントを投稿